Turing, Richards and Morphogenesis
Bernard Richards
Introduction
I first met Dr Alan Turing in early 1953 after I had just graduated with a degree in Mathematics. I was keen to do research for a Master’s Degree and, because I had studied Physics and Statistics, in addition to the standard Pure and Applied Mathematics within my Degree Course, a wide choice was available. But I had heard about this new electronic computer housed in an adjacent building. The Ferranti-built Mark I computer had been purchased by the Royal Society and installed in the newly-built Computing Laboratory of the University of Manchester in 1951.
I was introduced to Dr Tony Brooker, the Deputy Head of the Laboratory and Head of Programming, who told me about Turing’s achievements in the Laboratory. So when I met with Turing later that day I recognised that here was a genius, since having heard about his programming talents in that morning and his Morphogenesis work in that afternoon, he seemed to me to transcend both. I left that introductory meeting knowing that, whilst I had taken upon myself an intellectual challenge, I was embarking on a stimulating experience.
The task that was explained to me was that I must solve the Morphogenesis Equations for the spherical case. (See below.)
In the field of programming, Alan Turing had devised and produced the first ”Scheme” (analogous to today’s Operating Systems) for the Manchester Mark I Computer. It was called “Scheme A”. and significantly did two things, namely it took into the computer from 5-hole teleprinter paper-tape the program, and also carried out the task of transferring pages of program for the drum (the backing store) to the RAM high-speed store (cathode-ray tubes). This Scheme A was written in 1951 but was superseded in 1952 by Scheme B devised and written by Tony Brooker and Miss Cicely Popplewell. Perhaps not surprisingly, Turing never transferred to Scheme B but stayed with Scheme A all his life.
One day in late Summer, Turing saw me typing decimal numbers into the teleprinter to produce a data-tape for my program. When asked what I was doing typing decimal numbers, I explained to Turing that it was data for the coefficients in the equations. He said that I needed to convert my decimal numbers to base 32 for input into the computer via Scheme A. I told him that my route would work as I was using Scheme B which permitted input in decimal numbers. His response was a high-pitched “Oh”. Thereafter he never commented upon my programming techniques but used our times together to get excited about Morphogenesis.
The more I got to know Turing, the more I learnt about his many talents, in particular his continued prowess as an athlete and his not infrequent runs from his home to the Laboratory: some ten miles.
Turing, Richards, and Morphogenesis
So I set to work on seeking a solution to the Morphogenesis Equations on a sphere. The theory was that a spherical organism was subject to diffusion across its surface membrane by an alien substance, eg sea-water. The Equations were:
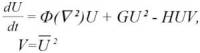
The function U, taken to be the radius vector from the centre to any point on the surface of the membrane, was argued to be representable as a series of Normalised Legendre Functions. The algebraic solution of the above equations ran to some 30 pages in my Thesis and are therefore not reproduced here. They are written in full in the book entitled “Morphogenesis” which is a tribute to Turing, edited by P. T. Saunders, published by North Holland, 1992.
The algebraic solution of the equations revealed a family of solutions, corresponding to a parameter n, taking values 2, 4. 6.
When I had solved the algebraic equations, I then used the computer to plot the shape of the resulting organisms. Turing told me that there were real organisms corresponding to what I had produced. He said that they were described and depicted in the records of the voyages of HMS Challenger in the 19th Century.
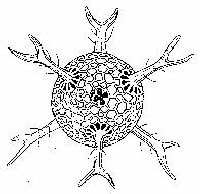
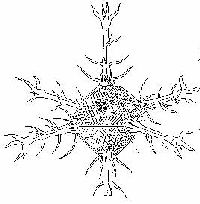
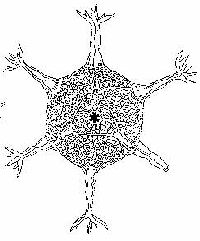
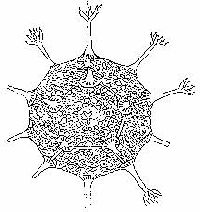
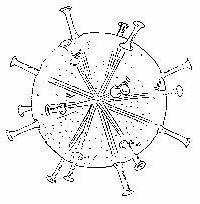
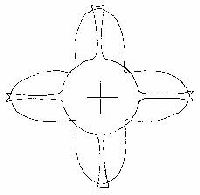
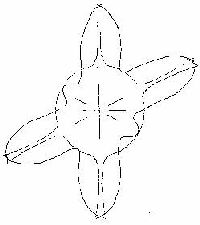
I solved the equations and produced a set of solutions which corresponded to the actual species of Radiolaria discovered by HMS Challenger in the 19th century. That expedition to the Pacific Ocean found eight variations in the growth patterns. These are shown in the following figures. The essential feature of the growth is the emergence of elongated "spines" protruding from the sphere at regular positions. Thus the species comprised two, six, twelve, and twenty, spine variations.
Thus, Figure 1 has two spines, one at the north pole and one at the south pole. Figure 2 shows a version with 6 spines. Figure 3 shows another species with 6 spines and Figure 4 has 12 spines. Figure 5 has 20 spines as does Figure 6.
Solving the Morphogenesis Equation produced theoretical solutions corresponding to the 2, 6, 12, and 20 spine variations. I then compared the shape of the computer solution with that of the actual creatures discovered by HMS Challenger. Figure 7 shows the solution for the 6 spine variant superimposed on the real 6 spine Radiolaria, the species Circopus Sexfarcus. The ratio of sphere to spine fits exactly. Figure 6 shows the fit for the 12 spine version, ie superimposing the computer solution upon Circogonia Icosahedra.
My work seemed to vindicate Turing's Theory of Morphogenesis. These results were obtained in late June of 1954: alas Alan Turing died on 7th June that year (still in his prime- aged 41), just a few days short of his birthday on the 23rd. Sadly, although he knew of the Radiolaria drawings from the Challenger voyage, he never saw the full outcome of my work nor indeed the accurate match between the computer results shown in Figures 7 and 8 and Radiolaria.